Physics:Elastomer

From HandWiki
Short description: Polymer with rubber-like elastic properties


An elastomer is a polymer with viscoelasticity (i.e. both viscosity and elasticity) and with weak intermolecular forces, generally low Young's modulus (E) and high failure strain compared with other materials.[1] The term, a portmanteau of elastic polymer,[2] is often used interchangeably with rubber, although the latter is preferred when referring to vulcanisates.[3] Each of the monomers which link to form the polymer is usually a compound of several elements among carbon, hydrogen, oxygen and silicon. Elastomers are amorphous polymers maintained above their glass transition temperature, so that considerable molecular reconformation is feasible without breaking of covalent bonds. At ambient temperatures, such rubbers are thus relatively compliant (E ≈ 3 MPa) and deformable. Their primary uses are for seals, adhesives and molded flexible parts.[citation needed]

IUPAC definition
Elastomer: Polymer that displays rubber-like elasticity[4]

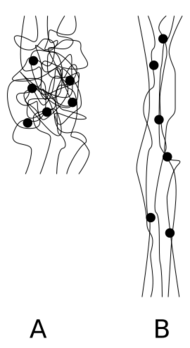
Rubber-like solids with elastic properties are called elastomers. Polymer chains are held together in these materials by relatively weak intermolecular bonds, which permit the polymers to stretch in response to macroscopic stresses.

(A) is an unstressed polymer; (B) is the same polymer under stress. When the stress is removed, it will return to the A configuration. (The dots represent cross-links)

Elastomers are usually thermosets (requiring vulcanization) but may also be thermoplastic (see thermoplastic elastomer). The long polymer chains cross-link during curing (i.e. vulcanizing). The molecular structure of elastomers can be imagined as a 'spaghetti and meatball' structure, with the meatballs signifying cross-links. The elasticity is derived from the ability of the long chains to reconfigure themselves to distribute an applied stress. The covalent cross-linkages ensure that the elastomer will return to its original configuration when the stress is removed.

Crosslinking most likely occurs in an equilibrated polymer without any solvent. The free energy expression derived from the Neohookean model of rubber elasticity is in terms of free energy change due to deformation per unit volume of the sample. The strand concentration, v, is the number of strands over the volume which does not depend on the overall size and shape of the elastomer.[5] Beta relates the end-to-end distance of polymer strands across crosslinks over polymers that obey random walk statistics.

[math]\displaystyle{ \Delta f_d = \frac{\Delta F_d}{V} = \frac{K_BT\nu_{el}\beta\lambda_1p^2 + \lambda_2p + 2\lambda_3p^2 - 3}{2} }[/math]

[math]\displaystyle{ v_{el} = \frac{n_{el}}{V} , \beta = 1 }[/math]

In the specific case of shear deformation, the elastomer besides abiding to the simplest model of rubber elasticity is also incompressible. For pure shear we relate the shear strain, to the extension ratios lambdas. Pure shear is a two-dimensional stress state making lambda equal to 1, reducing the energy strain function above to:

[math]\displaystyle{ \Delta f_{d}= \frac{k_{B}T\nu_{s}\beta\gamma^2}{2} }[/math]

To get shear stress, then the energy strain function is differentiated with respect to shear strain to get the shear modulus, G, times the shear strain:

[math]\displaystyle{ \sigma_{12} = \frac{d(\Delta f_{d})}{d\gamma} = G\gamma }[/math]

Shear stress is then proportional to the shear strain even at large strains.[6] Notice how a low shear modulus correlates to a low deformation strain energy density and vice versa. Shearing deformation in elastomers, require less energy to change shape than volume.

[math]\displaystyle{ \Delta f_d = W = \frac{G(\lambda_{1p}^2+\lambda_{2p}^2+\lambda_{3p}^2-3)}{2} }[/math]

Examples

Unsaturated rubbers that can be cured by sulfur vulcanization:

Saturated rubbers that cannot be cured by sulfur vulcanization:

Various other types of elastomers:

See also

References

  1. ↑ De, Sadhan K. (31 December 1996). Rubber Technologist's Handbook, Volume 1 (1st ed.). Smithers Rapra Press. p. 287. ISBN 978-1859572627. https://books.google.com/books?id=2rxFOm68Ui8C&q=elastomer+low+%22young%27s+modulus%22&pg=PA287. Retrieved 7 February 2017. 
  2. ↑ Gent, Alan N.. "Elastomer Chemical Compound". https://www.britannica.com/science/elastomer. 
  3. ↑ Alger, Mark (21 April 1989). Polymer Science Dictionary. Springer. p. 503. ISBN 1851662200. https://books.google.com/books?id=OSAaRwBXGuEC&q=rubber+term+preferred+vulcanisates&pg=PA503. Retrieved 7 February 2017. 
  4. ↑ Alemán, J. V.; Chadwick, A. V.; He, J.; Hess, M.; Horie, K.; Jones, R. G.; Kratochvíl, P.; Meisel, I. et al. (2007). "Definitions of Terms Relating to the Structure and Processing of Sols, Gels, Networks, and Inorganic–Organic Hybrid Materials (IUPAC Recommendations 2007)". Pure and Applied Chemistry 79 (10): 1801–1829. doi:10.1351/pac200779101801. https://www.iupac.org/publications/pac/pdf/2007/pdf/7910x1801.pdf. Retrieved 2017-07-14. 
  5. ↑ Boczkowska, Anna; Awietjan, Stefan F.; Pietrzko, Stanisław; Kurzydłowski, Krzysztof J. (2012-03-01). "Mechanical Properties of Magnetorheological Elastomers under Shear Deformation". Composites Part B: Engineering 43 (2): 636–640. doi:10.1016/j.compositesb.2011.08.026. ISSN 1359-8368. https://www.sciencedirect.com/science/article/pii/S135983681100360X. 
  6. ↑ Liao, Guojiang; Gong, Xinglong; Xuan, Shouhu (2013-09-01). "Influence of Shear Deformation on the Normal Force of Magnetorheological Elastomer". Materials Letters 106: 270–272. doi:10.1016/j.matlet.2013.05.035. ISSN 0167-577X. https://www.sciencedirect.com/science/article/pii/S0167577X13006836. 

External links



Retrieved from "https://handwiki.org/wiki/index.php?title=Physics:Elastomer&oldid=3285375"