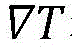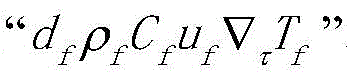CN116341195A - Deep crack-bedrock interbedded dry hot rock heat energy exploitation method - Google Patents
Deep crack-bedrock interbedded dry hot rock heat energy exploitation method Download PDFInfo
- Publication number
- CN116341195A CN116341195A CN202310082531.9A CN202310082531A CN116341195A CN 116341195 A CN116341195 A CN 116341195A CN 202310082531 A CN202310082531 A CN 202310082531A CN 116341195 A CN116341195 A CN 116341195A
- Authority
- CN
- China
- Prior art keywords
- heat
- rock
- temperature
- working medium
- ganre
- Prior art date
- Legal status (The legal status is an assumption and is not a legal conclusion. Google has not performed a legal analysis and makes no representation as to the accuracy of the status listed.)
- Pending
Links
- 239000011435 rock Substances 0.000 title claims abstract description 148
- 238000000034 method Methods 0.000 title claims abstract description 57
- 238000004519 manufacturing process Methods 0.000 claims abstract description 148
- 238000002347 injection Methods 0.000 claims abstract description 99
- 239000007924 injection Substances 0.000 claims abstract description 99
- 239000007787 solid Substances 0.000 claims abstract description 14
- XLYOFNOQVPJJNP-UHFFFAOYSA-N water Substances O XLYOFNOQVPJJNP-UHFFFAOYSA-N 0.000 claims abstract description 14
- 238000004088 simulation Methods 0.000 claims abstract description 13
- 238000012546 transfer Methods 0.000 claims abstract description 11
- 235000020681 well water Nutrition 0.000 claims description 28
- 239000002349 well water Substances 0.000 claims description 28
- 230000008859 change Effects 0.000 claims description 22
- 238000009826 distribution Methods 0.000 claims description 20
- 230000000694 effects Effects 0.000 claims description 12
- 238000010276 construction Methods 0.000 claims description 9
- 230000008569 process Effects 0.000 claims description 7
- 239000011159 matrix material Substances 0.000 claims description 6
- 238000004458 analytical method Methods 0.000 claims description 5
- 238000011835 investigation Methods 0.000 claims description 5
- 238000012544 monitoring process Methods 0.000 claims description 4
- 230000006835 compression Effects 0.000 claims description 3
- 238000007906 compression Methods 0.000 claims description 3
- 238000004134 energy conservation Methods 0.000 claims description 3
- 238000002474 experimental method Methods 0.000 claims description 3
- 239000007791 liquid phase Substances 0.000 claims description 3
- 230000035699 permeability Effects 0.000 claims description 3
- 239000012071 phase Substances 0.000 claims description 3
- 239000011148 porous material Substances 0.000 claims description 3
- 239000007790 solid phase Substances 0.000 claims description 3
- 238000011084 recovery Methods 0.000 claims description 2
- 239000000463 material Substances 0.000 claims 1
- 238000011161 development Methods 0.000 abstract description 14
- 238000003860 storage Methods 0.000 abstract description 5
- 206010017076 Fracture Diseases 0.000 description 60
- 239000010410 layer Substances 0.000 description 59
- 208000010392 Bone Fractures Diseases 0.000 description 56
- 238000005338 heat storage Methods 0.000 description 7
- 238000000605 extraction Methods 0.000 description 6
- 238000011160 research Methods 0.000 description 5
- 239000010438 granite Substances 0.000 description 4
- 239000011229 interlayer Substances 0.000 description 3
- 230000008878 coupling Effects 0.000 description 2
- 238000010168 coupling process Methods 0.000 description 2
- 238000005859 coupling reaction Methods 0.000 description 2
- 239000012530 fluid Substances 0.000 description 2
- 230000003993 interaction Effects 0.000 description 2
- 238000005065 mining Methods 0.000 description 2
- 238000005457 optimization Methods 0.000 description 2
- 238000009933 burial Methods 0.000 description 1
- 238000004891 communication Methods 0.000 description 1
- 230000001808 coupling effect Effects 0.000 description 1
- 238000001514 detection method Methods 0.000 description 1
- 238000005553 drilling Methods 0.000 description 1
- 238000011156 evaluation Methods 0.000 description 1
- 238000000265 homogenisation Methods 0.000 description 1
- 238000011065 in-situ storage Methods 0.000 description 1
- 238000005259 measurement Methods 0.000 description 1
- 238000012986 modification Methods 0.000 description 1
- 230000004048 modification Effects 0.000 description 1
- 230000002035 prolonged effect Effects 0.000 description 1
- 239000000243 solution Substances 0.000 description 1
- 239000000126 substance Substances 0.000 description 1
- 238000012360 testing method Methods 0.000 description 1
Images
Classifications
-
- E—FIXED CONSTRUCTIONS
- E21—EARTH OR ROCK DRILLING; MINING
- E21B—EARTH OR ROCK DRILLING; OBTAINING OIL, GAS, WATER, SOLUBLE OR MELTABLE MATERIALS OR A SLURRY OF MINERALS FROM WELLS
- E21B43/00—Methods or apparatus for obtaining oil, gas, water, soluble or meltable materials or a slurry of minerals from wells
-
- E—FIXED CONSTRUCTIONS
- E21—EARTH OR ROCK DRILLING; MINING
- E21B—EARTH OR ROCK DRILLING; OBTAINING OIL, GAS, WATER, SOLUBLE OR MELTABLE MATERIALS OR A SLURRY OF MINERALS FROM WELLS
- E21B49/00—Testing the nature of borehole walls; Formation testing; Methods or apparatus for obtaining samples of soil or well fluids, specially adapted to earth drilling or wells
-
- G—PHYSICS
- G06—COMPUTING; CALCULATING OR COUNTING
- G06F—ELECTRIC DIGITAL DATA PROCESSING
- G06F17/00—Digital computing or data processing equipment or methods, specially adapted for specific functions
- G06F17/10—Complex mathematical operations
- G06F17/11—Complex mathematical operations for solving equations, e.g. nonlinear equations, general mathematical optimization problems
-
- G—PHYSICS
- G06—COMPUTING; CALCULATING OR COUNTING
- G06F—ELECTRIC DIGITAL DATA PROCESSING
- G06F30/00—Computer-aided design [CAD]
- G06F30/20—Design optimisation, verification or simulation
-
- G—PHYSICS
- G06—COMPUTING; CALCULATING OR COUNTING
- G06F—ELECTRIC DIGITAL DATA PROCESSING
- G06F2113/00—Details relating to the application field
- G06F2113/08—Fluids
-
- G—PHYSICS
- G06—COMPUTING; CALCULATING OR COUNTING
- G06F—ELECTRIC DIGITAL DATA PROCESSING
- G06F2119/00—Details relating to the type or aim of the analysis or the optimisation
- G06F2119/08—Thermal analysis or thermal optimisation
-
- G—PHYSICS
- G06—COMPUTING; CALCULATING OR COUNTING
- G06F—ELECTRIC DIGITAL DATA PROCESSING
- G06F2119/00—Details relating to the type or aim of the analysis or the optimisation
- G06F2119/14—Force analysis or force optimisation, e.g. static or dynamic forces
-
- Y—GENERAL TAGGING OF NEW TECHNOLOGICAL DEVELOPMENTS; GENERAL TAGGING OF CROSS-SECTIONAL TECHNOLOGIES SPANNING OVER SEVERAL SECTIONS OF THE IPC; TECHNICAL SUBJECTS COVERED BY FORMER USPC CROSS-REFERENCE ART COLLECTIONS [XRACs] AND DIGESTS
- Y02—TECHNOLOGIES OR APPLICATIONS FOR MITIGATION OR ADAPTATION AGAINST CLIMATE CHANGE
- Y02E—REDUCTION OF GREENHOUSE GAS [GHG] EMISSIONS, RELATED TO ENERGY GENERATION, TRANSMISSION OR DISTRIBUTION
- Y02E10/00—Energy generation through renewable energy sources
- Y02E10/10—Geothermal energy
Landscapes
- Engineering & Computer Science (AREA)
- Physics & Mathematics (AREA)
- General Physics & Mathematics (AREA)
- Life Sciences & Earth Sciences (AREA)
- Theoretical Computer Science (AREA)
- Geology (AREA)
- Mining & Mineral Resources (AREA)
- Mathematical Physics (AREA)
- Mathematical Analysis (AREA)
- Pure & Applied Mathematics (AREA)
- Mathematical Optimization (AREA)
- Environmental & Geological Engineering (AREA)
- General Engineering & Computer Science (AREA)
- General Life Sciences & Earth Sciences (AREA)
- Fluid Mechanics (AREA)
- Computational Mathematics (AREA)
- Geochemistry & Mineralogy (AREA)
- Data Mining & Analysis (AREA)
- Evolutionary Computation (AREA)
- Operations Research (AREA)
- Geometry (AREA)
- Algebra (AREA)
- Computer Hardware Design (AREA)
- Databases & Information Systems (AREA)
- Software Systems (AREA)
- Management, Administration, Business Operations System, And Electronic Commerce (AREA)
Abstract
The invention relates to the technical field of deep geothermal energy development, in particular to a deep crack-bedrock interbedded dry hot rock thermal energy exploitation method, which comprises the following steps: constructing a discrete fracture network model, and revising the discrete fracture network model by considering porous medium heat transfer, darcy's law and solid mechanics, namely a heat-flow-solid physical field; respectively simulating three different injection and production modes of upper injection and lower production, lower injection and upper production and same-layer injection and production for the revised discrete fracture network model; and selecting an optimal injection and production mode and injection and production parameters of the crack-bedrock interbed developmental thermal storage according to the relation between the water production temperature of the production well and the injection speed, the injection temperature and the production pressure difference of the heat carrying working medium. According to the method, the discrete fracture network model is established to describe the dry hot rock reservoir in a vivid and detailed mode, and the method is closer to the dry hot rock reservoir in a real scene, so that the simulation result is more real and reliable.
Description
Technical Field
The invention relates to the technical field of deep geothermal energy development, in particular to a deep crack-bedrock interbedded dry hot rock thermal energy exploitation method.
Background
Dry hot rock (HDR) is an important geothermal resource, typically buried 3-10 km underground, containing no or little fluid and a temperature higher than 180 ℃ at a height Wen Yanti, where the stored heat is about 90% or more of the total geothermal energy.
The Enhanced Geothermal System (EGS) is characterized in that a low-temperature heat-carrying working medium is injected into a deep high-temperature dry-hot rock reservoir, and flows between injection and production wells through the working medium, so that the exploitation and utilization of geothermal energy are realized. The low-temperature heat-carrying working medium is in contact with the deep layer height Wen Yanti to generate heat exchange, and heat is transferred from the high-temperature dry-hot rock mass to the low-temperature heat-carrying working medium due to the fact that the temperature of the dry-hot rock reservoir is high, so that the injected heat-carrying working medium is heated through heat exchange. The deep geothermal energy extraction relates to the complex time-space evolution law of the hydrodynamic field, the stress field and the temperature field in the fractured rock body, namely the problem of heat-fluid-solid coupling. The method comprehensively considers the coupling effect between fields, and is closer to the geological condition of the dry hot rock reservoir under the real condition. Due to differences in geologic motion, in-situ stress conditions, petrography, rock mass frawability, etc., thermal reservoir rocks exhibit strong reservoir space heterogeneity at thermal reservoir site scale. Literature investigation shows that the existing deep geothermal energy development mode and construction parameter optimization are based on a single fracture geological model or a single bedrock geological model, the fracture characteristic parameters are subjected to homogenization and equivalence, and the influence of the fracture system density, the occurrence and the spatial communication relation on the heat exchange effect of the heat carrying working medium is difficult to embody. The demonstration of deep geothermal energy development mode of a interbed geological model based on a crack-bedrock symbiotic system is not disclosed. However, due to the complexity of natural and artificial cracks of the rock mass, the low-temperature heat-carrying working medium is injected into the plane to be extruded and developed along the dominant direction of the fracture network; in the longitudinal direction, a crack layer with high flow conductivity and a compact bedrock layer develop mutually, and a low-temperature front edge is a finger-in breakthrough after heat carrying working medium is injected, so that the method has obvious influence on the heat recovery rate and the service life of a geothermal field, and the research on the exploitation methods of different injection and extraction modes and the longitudinal mutually laminated development of the crack of a dry-hot rock reservoir is lacking.
The interrelation of the injection well and the production well in the hot dry rock reservoir can affect the heat extraction effect of the heat carrying medium in the hot dry rock. Because of different injection and production modes, the sweep range of the heat carrying working medium in the dry hot rock reservoir is also different, and the heat extraction effect of the heat carrying working medium in the dry hot rock reservoir is also different. At present, the type research of the well is relatively extensive, but the related research of the injection and production mode and the construction parameters based on the fracture-bedrock interbedded geothermal reservoir is still blank. Therefore, the development effect of the multi-physical field and crack interbed stack development of the geothermal reservoir under different injection and production modes still needs to be further researched, so that rich geothermal resources in China can be more efficiently developed and utilized.
A Chinese patent No. CN112984849B 'method for developing the karst heat storage of the chills and the crack type heat storage geothermal heat of the metamorphic rock stratum' discloses a method for developing the karst heat storage of the chills and the crack type heat storage geothermal heat of the metamorphic rock stratum. The method mainly solves the technical problems that the shallow karst heat storage and collection temperature is low, the influence on resident domestic water is generated, 100% recharging is not realized and the like in the geothermal process.
A Chinese patent No. CN115573692A discloses a system and a method for constructing a dry-hot rock artificial thermal reservoir, which are used for constructing a vertical fracture system, so that the difficulty of stratum fracturing is small and the fracturing range is wide.
The Chinese patent No. CN114429011A discloses a method, a device and a medium for calculating the total heat exchange surface of a dry-hot rock heat storage fracture. The invention establishes the relationship between the generating capacity of the ground system and the parameters of the underground heat storage fracturing artificial cracks. The patent is not directed to geothermal resource development techniques that take into account fracture-bedrock longitudinal heterogeneity and multiple physical field coupling.
None of the above patents considers the heterogeneity of the reservoir and fails to describe the fracture system geometry truly and effectively.
Disclosure of Invention
The invention aims to provide a method for exploiting heat energy of dry-heated rock by considering interaction among multiple physical fields, longitudinal stacking development of fracture layers and different injection and extraction modes, and aims to solve the problem of missing of the existing deep fracture-bedrock inter-layer type heat energy exploiting method of the dry-heated rock reservoir.
In order to achieve the above purpose, the invention adopts the following technical scheme: a deep crack-bedrock interbedded dry hot rock heat energy exploitation method comprises the following steps:
step one: and determining the reservoir temperature of the deep high-temperature dry hot rock reservoir and the distribution state and distribution form of cracks in the reservoir based on borehole core temperature monitoring, geological outcrop investigation, borehole core crack description and geophysical crack prediction results.
Step two: and (3) establishing a discrete fracture network model of the deep high Wen Ganre rock reservoir by using the existing software in the field according to the reservoir temperature of the deep high Wen Ganre rock reservoir obtained in the step one and the distribution state and the distribution form of the fractures in the reservoir, wherein the fracture layer and the bedrock layer longitudinally and multiply overlap and develop.
Step three: considering porous medium heat transfer, darcy's law and solid mechanics, namely heat-flow-solid physical field, revising the discrete fracture network model, wherein the revising process is as follows:
the flow of the heat carrying working medium in the discrete fracture network model is described by mass conservation law (2-1):
wherein ρ is f Is a heat-carrying working mediumDensity, epsilon p Porosity of the deep high Wen Ganre rock, t is simulation time, Q m The method is characterized in that the method is a mass source in deep high Wen Ganre rock, and u is the flow velocity of a heat carrying working medium in deep high Wen Ganre rock;
the motion of the heat carrying working medium is described by Darcy's law (3-1):
wherein k is the permeability of the deep high Wen Ganre rock, mu is the viscosity of the heat carrying working medium,for pressure gradient +.>Is a deep high Wen Ganre rock reservoir depth gradient.
The porous medium heat transfer process in the discrete fracture network model is described by an energy conservation equation (4-1):
wherein (ρC) p ) eff =ε p ρ f +(1-ε p )ρ s C s ,λ eff =ε p λ f +(1-ε p )λ s 。(ρC p ) eff And lambda (lambda) eff The method comprises the steps of respectively obtaining effective heat capacity and effective heat conductivity coefficient through volume average, wherein T is the reservoir temperature of the deep-layer high Wen Ganre rock, ρ is the density of the deep-layer high Wen Ganre rock, C is the specific heat capacity, λ is the heat conductivity coefficient, and subscripts "s" and "f" respectively represent a solid phase and a liquid phase; c (C) p Constant pressure specific heat capacity of deep high Wen Ganre rock, C p,f The specific heat capacity of the heat carrying working medium in the deep high Wen Ganre rock,representing the temperature gradient of the deep Wen Ganre rock;
the mechanical deformation of the deep-layer high Wen Ganre rock is based on the heat-hole-elasticity principle, and the control equation (5-1) for matrix deformation is obtained by combining the effects of thermal expansion and pore pressurization, wherein the control equation is as follows:
wherein sigma' ij Is effective stress, sigma ij For normal phase compression stress ε ij Is strain, E is Young's modulus, v is Poisson's ratio, α B Is the specific Australian coefficient, p is the pressure of the heat-carrying working medium, delta ij Is a Cronecker function, alpha T Is the coefficient of thermal expansion, Δt is the temperature increment, Δt=t-T ref T and T ref The temperature of the deep Wen Ganre rock and the initial reference temperature of the injected heat carrying working medium are respectively;
the temperature field equation (6-1) for deep high Wen Ganre rock is:
wherein ρ is s Is a deep high Wen Ganre rock density; lambda (lambda) s The heat conduction coefficient of the rock with deep height Wen Ganre; cs is the specific heat capacity of deep high Wen Ganre rock;is the Laplace operator, which is the divergence of the gradient; t (T) s Is the surface temperature of the deep high Wen Ganre rock; w is a heat source.
Crack water temperature field equation (7-1):
wherein d f Is the crack opening degree; ρ f The density of the working medium carrying heat; c (C) f The specific heat capacity of the heat carrying working medium; t (T) f The temperature of the working medium carrying heat; u (u) f Is the flow velocity of the heat carrying working medium; lambda (lambda) f Is the heat conduction coefficient of the heat carrying working medium;is derived tangentially along the crack; u (u) f The flow velocity of the heat carrying working medium in the crack; t (T) f The temperature of the heat carrying working medium in the crack; w (W) f The heat absorbed by the heat carrying working medium on the surface of the crack from the deep high Wen Ganre rock.
The heat exchange between the heat carrying working medium in the crack and the deep high-temperature dry hot rock obeys Newton heat exchange formula, and the heat exchange quantity of the heat carrying working medium and the deep high-temperature dry hot rock at the boundary is calculated by using convection heat transfer coefficients, namely the average temperature of the heat carrying working medium in the crack is considered to be different from the surface temperature of the deep high-temperature dry hot rock, and the heat (8-1) transferred from the deep high Wen Ganre rock to the heat carrying working medium in the crack in unit area is as follows:
W f =h(T s -T f ) (8-1)
wherein h is the heat exchange coefficient, T s Is the surface temperature of the deep high Wen Ganre rock.
Step four: and respectively adopting three different injection and production modes of upper injection and lower production, lower injection and upper production and same-layer injection and production for the discrete fracture network model after the revision of the step three.
Step five: three injection and production modes proposed in the fourth step are adopted for the revised discrete fracture network model, and the injection speed q of the heat carrying working medium is changed for each injection and production mode inj Injection temperature T inj And the production pressure difference delta P, the production well water temperature is obtained along with q in the production time by a numerical simulation method inj 、T inj And as a result of delta P change, drawing a temperature change curve of the heat carrying working medium at the outlet end of the production well by taking the exploitation time of the heat energy of the deep layer high Wen Ganre rock as an abscissa and the water production temperature of the production well as an ordinate, thereby obtaining the temperature T of the heat carrying working medium at the outlet end of the production well out Is a law of variation of (c).
Step six: setting injection rate q inj Injection temperature T inj Different values of the construction parameters of the production pressure difference delta P are adopted, and an orthogonal experimental method is applied to establish N sets of construction parameter combination experimental schemes;
according to the method of the fifth step, N production well water temperature change curves are obtained, the N production well water temperature change curves are analyzed by applying a range analysis method, an optimal production well water temperature change curve is selected, and heat energy exploitation is carried out on the target dry hot rock reservoir according to technological parameters represented by the optimal production well water temperature change curve.
Preferably, when the discrete fracture network model is built in the second step, the fracture is regarded as a linear unit without thickness, the deep high-temperature dry-hot rock is discrete by using a solid unit, wherein the fracture length distribution of the fracture network generally adopts a power law distribution model, and the relation between the number of the fractures and the length is expressed as (1-1):
n(l,L)=αL D l -a ,l∈[l min ,l max ] (1-1)
wherein L is the domain size; l is the crack length; n (L, L) is the length L of the fracture in the square reservoir with the side length L being [ L, l+dl ]]Number of cracks in (dl < l); d is a fractal dimension; alpha is a crack length index; alpha is a constant related to fracture density; l (L) max And l min The maximum and minimum values of fracture length in a square reservoir, respectively.
Compared with the prior art, the method has the following advantages:
1. the invention fully discusses the advantages and disadvantages of developing the heat energy of the deep dry hot rock reservoir by different injection and production modes, optimizes reasonable injection and production parameters by combining the water temperature of the production well, and provides a new thought for the heat energy development of the subsequent deep dry hot rock reservoir.
2. The invention considers the interaction among three different physical fields of heat, fluid and solid, solves the pain point and the difficulty of deep dry hot rock reservoir development, and realizes the research of dry hot rock injection and production mode optimization.
3. According to the method, the discrete fracture network model is established to describe the dry hot rock reservoir in a vivid and detailed mode, and the method is closer to the dry hot rock reservoir in a real scene, so that the simulation result is more real and reliable.
Drawings
FIG. 1 is a flow chart of the method of the present invention.
FIG. 2 is a fracture-bedrock interbedded dry thermal rock geologic model of the up-injection and down-production mode.
FIG. 3 is a fracture-bedrock interbedded dry-hot rock geologic model of a lower-stream, upper-production mode.
Fig. 4 is a fracture-bedrock interbedded dry-hot rock geologic model of the same-layer injection and production mode.
FIG. 5 is a graph showing the temperature relationship of the production well water after 20 years of production in the three injection and production modes respectively in the embodiment.
FIG. 6 is a graph showing the temperature relationship of the production well water after 20 years of exploitation in the same-layer injection and production mode under different mass flow rates of the heat-carrying working medium in the embodiment.
FIG. 7 is a graph showing the temperature relationship of the production well water after 20 years of exploitation in the same-layer injection-production mode at different injection temperatures of the heat-carrying working medium in the embodiment.
FIG. 8 is a graph showing the temperature relationship of the production water of the production well after 20 years of production in the same-layer injection-production mode under different production pressure differences in the embodiment.
Detailed Description
The following describes the scheme of the invention in detail with reference to the accompanying drawings.
Referring to fig. 1-4, a deep fracture-bedrock interbedded dry hot rock heat energy exploitation method comprises the following steps:
step one: determining the reservoir temperature of the deep high-temperature dry hot rock reservoir and the distribution state and distribution form of cracks in the reservoir based on borehole core temperature monitoring, geological outcrop investigation, borehole core crack description and geophysical crack prediction results; the method comprises the steps of determining the reservoir temperature of a deep high-temperature dry hot rock reservoir and the distribution state and distribution form of cracks in the reservoir through borehole core temperature monitoring, geological outcrop investigation, borehole core crack description and geophysical crack prediction results, and in addition, the deep high Wen Ganre rock refers to deep crack-bedrock inter-layer dry hot rock.
Step two: and (3) establishing a discrete fracture network model of the deep high Wen Ganre rock reservoir by using the existing software in the field according to the reservoir temperature of the deep high Wen Ganre rock reservoir obtained in the step one and the distribution state and the distribution form of the fractures in the reservoir, wherein the fracture layer and the bedrock layer longitudinally and multiply overlap and develop. The position, trend and opening of the crack are described according to actual conditions, namely, the crack is expressed by a discrete crack method; a discrete fracture network model of the deep high Wen Ganre rock reservoir was built using Matlab software.
Step three: considering porous medium heat transfer, darcy's law and solid mechanics, namely heat-flow-solid physical field, revising the discrete fracture network model, wherein the revising process is as follows: the discrete fracture network model performs heat exchange with the rock matrix established by the model by taking water as a heat carrying working medium, so as to extract heat.
The flow of the heat carrying working medium in the discrete fracture network model is described by mass conservation law (2-1):
wherein ρ is f For the density of the heat-carrying working medium epsilon p Porosity of the deep high Wen Ganre rock, t is simulation time, Q m The method is characterized in that the method is a mass source in deep high Wen Ganre rock, and u is the flow velocity of a heat carrying working medium in deep high Wen Ganre rock;
the motion of the heat carrying working medium is described by Darcy's law (3-1):
wherein k is the permeability of the deep high Wen Ganre rock, mu is the viscosity of the heat carrying working medium,for pressure gradient +.>Is a deep high Wen Ganre rock reservoir depth gradient.
The porous medium heat transfer process in the discrete fracture network model is described by an energy conservation equation (4-1):
wherein (ρC) p ) eff =ε p ρ f +(1-ε p )ρ s C s ,λ eff =ε p λ f +(1-ε p )λ s 。(ρC p ) eff And lambda (lambda) eff The method comprises the steps of respectively obtaining effective heat capacity and effective heat conductivity coefficient through volume average, wherein T is the reservoir temperature of the deep-layer high Wen Ganre rock, ρ is the density of the deep-layer high Wen Ganre rock, C is the specific heat capacity, λ is the heat conductivity coefficient, and subscripts "s" and "f" respectively represent a solid phase and a liquid phase; c (C) p Constant pressure specific heat capacity of deep high Wen Ganre rock, C p,f The specific heat capacity of the heat carrying working medium in the deep high Wen Ganre rock,representing the temperature gradient of the deep Wen Ganre rock.
The mechanical deformation of the deep-layer high Wen Ganre rock is based on the heat-hole-elasticity principle, and the control equation (5-1) for matrix deformation is obtained by combining the effects of thermal expansion and pore pressurization, wherein the control equation is as follows:
wherein sigma' ij Is effective stress, sigma ij For normal phase compression stress ε ij Is strain, E is Young's modulus, v is Poisson's ratio, α B Is the specific Australian coefficient, p is the pressure of the heat-carrying working medium, delta ij Is a Cronecker function, alpha T Is the coefficient of thermal expansion, Δt is the temperature increment, Δt=t-T ref T and T ref The temperature of the deep-layer high Wen Ganre rock and the initial reference temperature of the injected heat carrying working medium are respectively.
The temperature field equation (6-1) for deep high Wen Ganre rock is:
wherein ρ is s Is a deep high Wen Ganre rock density; lambda (lambda) s The heat conduction coefficient of the rock with deep height Wen Ganre; cs is the specific heat capacity of deep high Wen Ganre rock;is the Laplace operator, which is the divergence of the gradient; t (T) s Is the surface temperature of the deep high Wen Ganre rock; w is a heat source.
Crack water temperature field equation (7-1):
wherein d f Is the crack opening degree; ρ f The density of the working medium carrying heat; c (C) f The specific heat capacity of the heat carrying working medium; t (T) f The temperature of the working medium carrying heat; u (u) f Is the flow velocity of the heat carrying working medium; lambda (lambda) f Is the heat conduction coefficient of the heat carrying working medium;is derived tangentially along the crack; u (u) f The flow velocity of the heat carrying working medium in the crack; t (T) f The temperature of the heat carrying working medium in the crack; w (W) f The heat absorbed by the heat carrying working medium on the surface of the crack from the deep high Wen Ganre rock; second item->Indicating the convective effect of the heat carrying substance in the crack on the temperature field.
The heat exchange between the heat carrying working medium in the crack and the deep high-temperature dry hot rock obeys Newton heat exchange formula, and the heat exchange quantity of the heat carrying working medium and the deep high-temperature dry hot rock at the boundary is calculated by using convection heat transfer coefficients, namely the average temperature of the heat carrying working medium in the crack is considered to be different from the surface temperature of the deep high-temperature dry hot rock, and the heat (8-1) transferred from the deep high Wen Ganre rock to the heat carrying working medium in the crack in unit area is as follows:
W f =h(T s -T f ) (8-1)
wherein h is the heat exchange coefficient, T s Is the surface temperature of the deep high Wen Ganre rock.
Step four: under the influence of the space heterogeneity and the high diversion effect of the cracks, when the deep high Wen Ganre rock reservoir layer with the crack layer-bedrock layer developing mutually is injected and heated, the front edge of the low-temperature heat-carrying working medium is unevenly pushed in the plane and the longitudinal direction, so that part of production well sections are broken through prematurely, and the heat collection efficiency is influenced. Aiming at the engineering difficulty, based on the discrete fracture network model constructed in the step three, respectively adopting three different injection and production modes of upper injection and lower production, lower injection and upper production and same-layer injection and production to simulate the discrete fracture network model revised in the step three; taking three layers of crack stacking development as an example, dividing a crack layer into an upper layer, a middle layer and a lower layer, wherein three different injection and production modes are respectively as follows: upper and lower injection and upper production and same layer injection and production. The upper injection and lower production are carried out by taking the left end of the upper fracture layer as an injection end, namely an injection well, and the right end of the lower fracture layer as an outlet end, namely a production well; the lower injection production model takes the right end of the upper fracture layer as an outlet end, namely a production well, and the left end of the lower fracture layer as an injection end, namely an injection well; the same-layer injection and production model uses the left end of a dry-hot rock reservoir, namely the left end of an upper layer crack, a middle layer crack and a lower layer crack, the left end of a reservoir matrix rock body as an injection end, namely an injection well, and the right end of the reservoir matrix rock body as an outlet end, namely a production well.
Step five: three injection and production modes proposed in the fourth step are adopted for the revised discrete fracture network model, and the injection speed q of the heat carrying working medium is changed for each injection and production mode inj Injection temperature T inj And the production pressure difference delta P, the production well water temperature is obtained along with q in the production time by a numerical simulation method inj 、T inj And as a result of delta P change, drawing a temperature change curve of the heat carrying working medium at the outlet end of the production well by taking the exploitation time of the heat energy of the deep layer high Wen Ganre rock as an abscissa and the water production temperature of the production well as an ordinate, thereby obtaining the temperature T of the heat carrying working medium at the outlet end of the production well out Is a law of variation of (a); i.e. for an injection-production mode, the injection speed q inj Injection temperature T inj And the production pressure difference delta P is used as the input of a revised discrete fracture network model, and the output of the revised discrete fracture network model is the production well water temperature under the injection and production mode, and the q is continuously changed inj 、T inj And delta P, obtaining the corresponding production well water temperature, and drawing a temperature change curve of the heat carrying working medium at the outlet end of the production well according to the obtained production well water temperature; the same method is adopted for the other two injection and production modes.
According to the initial temperature, thermal storage thickness, stacking layer number, crack density and injection and production well distance of the target dry and hot rock reservoir, construction parameters are mainly optimized aiming at two aspects of an injection well and a production well, and the parameters to be optimized for the injection well are injection speed q inj Injection temperature T inj The production pressure differential Δp is primarily optimized for the production well. Finally, the water temperature T of the production well is used for out As an evaluation index of the merits of geothermal development effect.
Step six: setting injection rate q inj Injection temperature T inj And the production pressure difference delta P construction parameters are different in value, and an orthogonal experimental method is applied to establish N sets of construction parameter combination test schemes.
According to the method of the fifth step, N production well water temperature change curves are obtained, the N production well water temperature change curves are analyzed by applying a range analysis method, an optimal production well water temperature change curve is selected, and heat energy exploitation is carried out on the target dry hot rock reservoir according to technological parameters represented by the optimal production well water temperature change curve.
Specifically, when the discrete fracture network model is built in the second step, the fracture is regarded as a linear unit without thickness, the deep high-temperature dry-hot rock is discrete by using a solid unit, wherein the fracture length distribution of the fracture network generally adopts a power law distribution model, and the relation between the number of the fractures and the length is expressed as (1-1):
n(l,L)=αL D l -a ,l∈[l min ,l max ] (1-1)
wherein L is the domain size; l is the crack length; n (L, L) is the length L of the fracture in the square reservoir with the side length L being [ L, l+dl ]]Number of cracks in (dl < l); d is a fractal dimension; alpha is a crack length index; alpha is a constant related to fracture density; l (L) max And l min The maximum and minimum values of fracture length in a square reservoir, respectively.
Example 1:
the method takes a non-Bangor basin H-block granite down-the-road thermal field as an object, and according to seismic interpretation, well logging analysis and geological comprehensive research, the target thermal storage is a granite crack down-the-road dry thermal rock reservoir, the thermal storage burial depth ranges from 2800m to 3340m, the crack layer and the bedrock layer develop from an unconformity surface to a down-the-road inner curtain to form a mutual layer, and the method is typical crack-bedrock mutual layer thermal storage and has extremely strong longitudinal heterogeneity. The well drilling temperature actual measurement and the core description show that the bottom hole temperature of the dry-hot rock reservoir is 260-290 ℃, the average length of cracks is 12 m, and the inclination angle of the cracks is 20-80 degrees. The geothermal Tian Zhonggan hot rock reservoir cracks develop in a layered and superposed manner, and the crack layers are divided into an upper layer, a middle layer and a lower layer.
And (3) carrying out geothermal exploitation on the non-Bangor basin H block granite buried hill thermal field according to the analysis result, wherein the specific exploitation steps are as follows:
step one: and establishing a discrete fracture network model by adopting a numerical simulation method according to the relevant parameters of the dry-hot rock reservoir, wherein the length of the model is 3000m, and the width is 1240m. The average length of the crack is 8 meters, the inclination angle of the crack is 20-80 degrees, the average value is 65 degrees, and the crack layer is divided into an upper layer, a middle layer and a lower layer. The detection shows that the initial temperature of the dry-hot rock reservoir is 270 ℃, and the water with the initial temperature of 20 ℃ is taken as a low-temperature heat carrying medium to extract heat energy from the dry-hot rock reservoir.
Step two: the porous medium heat transfer, darcy's law and solid mechanics are considered for the physical model of the longitudinal superposition of the fracture layer and the bedrock layer.
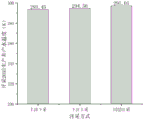
Step three: and respectively adopting three injection and production modes of upper injection and lower production, lower injection and upper production and same-layer injection and production, and establishing different injection and production mode models based on the discrete fracture network model. The three injection and production modes all predict the production well water temperature T under the 20 years of heat production period out As shown in fig. 5, the higher the temperature, the more sufficient the heat-carrying medium exchanges heat with the high Wen Yanti, and the better the heat collecting effect. As can be seen from fig. 5, the production well water temperature of the upper injection and lower production model is 293.45K after 20 years of operation, the production well water temperature of the lower injection and upper production model is 294.56K after 20 years of operation, and the production well water temperature of the same-layer injection and production model is 297.05K after 20 years of operation. Therefore, the dry-hot rock reservoir is suitable for the development mode of same-layer injection and production, namely, the injection well is positioned at one end of the dry-hot rock reservoir, the left end is set at the position, and the production well is positioned at the other end of the dry-hot rock reservoir, and the right end is set at the position.
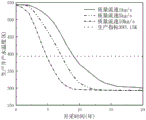
Step four: the dry hot rock reservoir is developed based on the same-layer injection and production mode preferred in the third step, the mining life of the dry hot rock reservoir is set to be 20 years, heat-carrying working media with the temperature of 20 ℃ are injected at mass flow rates of 1kg/s, 5kg/s and 10kg/s respectively, and temperature change curves (figure 6) of the heat-carrying working media at the outlet end of a production well are obtained under different injection speeds when the injection and production pressure difference is 5MPa through a numerical simulation method and the mining life is set to be 20 years. When the other parameters are the same, the mass flow rate of the heat carrying working medium is 8kg/s, and the injection and production pressure difference is 5MPa, the injection temperature of the heat carrying working medium is changed to be 10 ℃, 15 ℃ and 20 ℃, and the temperature change curve of the outlet end of the production well is obtained under the injection temperature of different heat carrying working mediums by a numerical simulation method (figure 7). Under the same conditions as the rest conditions, when the injection temperature of the heat carrying working medium is 20 ℃ and the mass flow rate of the heat carrying working medium is 8kg/s, the heat energy exploitation is carried out on the dry hot rock reservoir layer by using the production pressure differences of 4MPa, 6MPa and 8 MPa. And obtaining temperature change curves (figure 8) of the thermal-carrying working medium at the outlet end of the production well within 20 years under different pressure differences by a numerical simulation method.
Step five: the temperature of the heat carrying working medium at the outlet end of the production well and the injection speed q of the heat carrying working medium are obtained according to the numerical simulation result inj Injection temperature T inj And the production pressure difference DeltaP, FIG. 6 shows that if T is out >393.15K is a production index, when the mass flow rate of the heat carrying working medium is 1kg/s, 5kg/s and 10kg/s, the operation life of the system is 9 years, 7 years and 5 years respectively, the operation life of the system with the mass flow rate of 1kg/s is prolonged by 4 years compared with the operation life of the system with the mass flow rate of 10kg/s, and when the mass flow rate of the heat carrying working medium is 1kg/s, the system has higher production well water temperature than the other two types of production well water temperature at any moment. As can be seen from fig. 7, when the injection temperature of the heat-carrying working medium is 10 ℃, 15 ℃ and 20 ℃, the operation life of the system is 5 years, 7 years and 8 years respectively, and when the injection temperature of the heat-carrying working medium is 20 ℃, the operation life of the system is longest, and after 20 years of exploitation, the production well water temperature is highest compared with the other two types. FIG. 8 shows that the system operates under production pressure differences of 4MPa, 6MPa and 8MPaThe service life is 8 years, 6 years and 4 years respectively, namely the system has the maximum service life of 8 years when the production pressure difference of the production well is 4MPa, and the temperature of the produced water of the production well is high after 20 years of exploitation compared with the temperature of the production well when the production pressure difference is 6MPa and 8 MPa.
The result shows that when the injection speed of the heat carrying working medium is 1kg/s, the injection temperature of the heat carrying working medium is 20 ℃, the production pressure difference of the production well is 4MPa, the heat energy extraction effect of the crack-bedrock inter-layer type dry hot rock reservoir is best, and the parameters are the optimal injection and production parameters of the granite subsurface geothermal field.
The above embodiments are only for illustrating the present invention, wherein the implementation steps and related parameters can be changed, and all equivalent changes and modifications performed on the basis of the technical solution of the present invention should not be excluded from the protection scope of the present invention.
Claims (2)
1. A deep crack-bedrock interbedded dry hot rock heat energy exploitation method is characterized in that: the method comprises the following steps:
step one: determining the reservoir temperature of the deep high-temperature dry hot rock reservoir and the distribution state and distribution form of cracks in the reservoir based on borehole core temperature monitoring, geological outcrop investigation, borehole core crack description and geophysical crack prediction results;
step two: and (3) establishing a discrete fracture network model of the deep high Wen Ganre rock reservoir by using the existing software in the field according to the reservoir temperature of the deep high Wen Ganre rock reservoir obtained in the step one and the distribution state and the distribution form of the fractures in the reservoir, wherein the fracture layer and the bedrock layer longitudinally and multiply overlap and develop.
Step three: considering porous medium heat transfer, darcy's law and solid mechanics, namely heat-flow-solid physical field, revising the discrete fracture network model, wherein the revising process is as follows:
the flow of the heat carrying working medium in the discrete fracture network model is described by mass conservation law (2-1):
wherein ρ is f For the density of the heat-carrying working medium epsilon p Porosity of the deep high Wen Ganre rock, t is simulation time, Q m The method is characterized in that the method is a mass source in deep high Wen Ganre rock, and u is the flow velocity of a heat carrying working medium in deep high Wen Ganre rock;
the motion of the heat carrying working medium is described by Darcy's law (3-1):
wherein k is the permeability of the deep high Wen Ganre rock, mu is the viscosity of the heat carrying working medium,for pressure gradient +.>Is a deep high Wen Ganre rock reservoir depth gradient.
The porous medium heat transfer process in the discrete fracture network model is described by an energy conservation equation (4-1):
wherein (ρC) p ) eff =ε p ρ f +(1-ε p )ρ s C s ,λ eff =ε p λ f +(1-ε p )λ s 。(ρC p ) eff And lambda (lambda) eff The method comprises the steps of respectively obtaining effective heat capacity and effective heat conductivity coefficient through volume average, wherein T is the reservoir temperature of the deep-layer high Wen Ganre rock, ρ is the density of the deep-layer high Wen Ganre rock, C is the specific heat capacity, λ is the heat conductivity coefficient, and subscripts "s" and "f" respectively represent a solid phase and a liquid phase; c (C) p Constant pressure specific heat capacity of deep high Wen Ganre rock, C p,f Is a heat carrying working medium in a deep high Wen Ganre rockThe specific heat capacity of the material,representing the temperature gradient of the deep Wen Ganre rock;
the mechanical deformation of the deep-layer high Wen Ganre rock is based on the heat-hole-elasticity principle, and the control equation (5-1) for matrix deformation is obtained by combining the effects of thermal expansion and pore pressurization, wherein the control equation is as follows:
wherein sigma' ij Is effective stress, sigma ij For normal phase compression stress ε ij Is strain, E is Young's modulus, v is Poisson's ratio, α B Is the specific Australian coefficient, p is the pressure of the heat-carrying working medium, delta ij Is a Cronecker function, alpha T Is the coefficient of thermal expansion, Δt is the temperature increment, Δt=t-T ref T and T ref The temperature of the deep Wen Ganre rock and the initial reference temperature of the injected heat carrying working medium are respectively;
the temperature field equation (6-1) for deep high Wen Ganre rock is:
wherein ρ is s Is a deep high Wen Ganre rock density; lambda (lambda) s The heat conduction coefficient of the rock with deep height Wen Ganre; cs is the specific heat capacity of deep high Wen Ganre rock;is the Laplace operator, which is the divergence of the gradient; t (T) s Is the surface temperature of the deep high Wen Ganre rock; w is a heat source.
Crack water temperature field equation (7-1):
wherein d f Is the crack opening degree; ρ f The density of the working medium carrying heat; c (C) f The specific heat capacity of the heat carrying working medium; t (T) f The temperature of the working medium carrying heat; u (u) f Is the flow velocity of the heat carrying working medium; lambda (lambda) f Is the heat conduction coefficient of the heat carrying working medium;is derived tangentially along the crack; u (u) f The flow velocity of the heat carrying working medium in the crack; t (T) f The temperature of the heat carrying working medium in the crack; w (W) f The heat absorbed by the heat carrying working medium on the surface of the crack from the deep high Wen Ganre rock;
the heat exchange between the heat carrying working medium in the crack and the deep high-temperature dry hot rock obeys Newton heat exchange formula, and the heat exchange quantity of the heat carrying working medium and the deep high-temperature dry hot rock at the boundary is calculated by using convection heat transfer coefficients, namely the average temperature of the heat carrying working medium in the crack is considered to be different from the surface temperature of the deep high-temperature dry hot rock, and the heat (8-1) transferred from the deep high Wen Ganre rock to the heat carrying working medium in the crack in unit area is as follows:
W f =h(T s -T f )(8-1)
wherein h is the heat exchange coefficient, T s Is the surface temperature of the deep high Wen Ganre rock.
Step four: respectively adopting three different injection and production modes of upper injection and lower production, lower injection and upper production and same-layer injection and production for the discrete fracture network model after the revision of the step three;
step five: three injection and production modes proposed in the fourth step are adopted for the revised discrete fracture network model, and the injection speed q of the heat carrying working medium is changed for each injection and production mode inj Injection temperature T inj And the production pressure difference delta P, the production well water temperature is obtained along with q in the production time by a numerical simulation method inj 、T inj And as a result of delta P change, drawing a temperature change curve of the heat carrying working medium at the outlet end of the production well by taking the exploitation time of the heat energy of the deep layer high Wen Ganre rock as an abscissa and the water production temperature of the production well as an ordinate, thereby obtaining the temperature T of the heat carrying working medium at the outlet end of the production well out Law of variation of (a);
Step six: setting injection rate q inj Injection temperature T inj Different values of the construction parameters of the production pressure difference delta P are adopted, and an orthogonal experimental method is applied to establish N sets of construction parameter combination experimental schemes;
according to the method of the fifth step, N production well water temperature change curves are obtained, the N production well water temperature change curves are analyzed by applying a range analysis method, an optimal production well water temperature change curve is selected, and heat energy exploitation is carried out on the target dry hot rock reservoir according to technological parameters represented by the optimal production well water temperature change curve.
2. The deep fracture-bedrock interbedded dry thermal rock thermal energy recovery method of claim 1, wherein: when the discrete fracture network model is built in the second step, the fracture is regarded as a linear unit without thickness, the deep high-temperature dry-hot rock is discrete by using a solid unit, wherein the fracture length distribution of the fracture network generally adopts a power law distribution model, and the relation between the number of the fractures and the length is expressed as (1-1):
n(l,L)=αL D l -a ,l∈[l min ,l max ] (1-1)
wherein L is the domain size; l is the crack length; n (L, L) is the length L of the fracture in the square reservoir with the side length L being [ L, l+dl ]]Number of cracks in (dl < l); d is a fractal dimension; alpha is a crack length index; alpha is a constant related to fracture density; l (L) max And l min The maximum and minimum values of fracture length in a square reservoir, respectively.
Priority Applications (1)
| Application Number | Priority Date | Filing Date | Title |
|---|---|---|---|
| CN202310082531.9A CN116341195A (en) | 2023-02-08 | 2023-02-08 | Deep crack-bedrock interbedded dry hot rock heat energy exploitation method |
Applications Claiming Priority (1)
| Application Number | Priority Date | Filing Date | Title |
|---|---|---|---|
| CN202310082531.9A CN116341195A (en) | 2023-02-08 | 2023-02-08 | Deep crack-bedrock interbedded dry hot rock heat energy exploitation method |
Publications (1)
| Publication Number | Publication Date |
|---|---|
| CN116341195A true CN116341195A (en) | 2023-06-27 |
Family
ID=86881232
Family Applications (1)
| Application Number | Title | Priority Date | Filing Date |
|---|---|---|---|
| CN202310082531.9A Pending CN116341195A (en) | 2023-02-08 | 2023-02-08 | Deep crack-bedrock interbedded dry hot rock heat energy exploitation method |
Country Status (1)
| Country | Link |
|---|---|
| CN (1) | CN116341195A (en) |
Cited By (1)
| Publication number | Priority date | Publication date | Assignee | Title |
|---|---|---|---|---|
| CN116882221A (en) * | 2023-09-08 | 2023-10-13 | 山东科技大学 | Geothermal exploitation numerical simulation method and system based on three-dimensional fracture type thermal storage model |
-
2023
- 2023-02-08 CN CN202310082531.9A patent/CN116341195A/en active Pending
Cited By (2)
| Publication number | Priority date | Publication date | Assignee | Title |
|---|---|---|---|---|
| CN116882221A (en) * | 2023-09-08 | 2023-10-13 | 山东科技大学 | Geothermal exploitation numerical simulation method and system based on three-dimensional fracture type thermal storage model |
| CN116882221B (en) * | 2023-09-08 | 2023-12-01 | 山东科技大学 | Geothermal exploitation numerical simulation method and system based on three-dimensional fracture type thermal storage model |
Similar Documents
| Publication | Publication Date | Title |
|---|---|---|
| Zhang et al. | Performance analysis of a downhole coaxial heat exchanger geothermal system with various working fluids | |
| Zeng et al. | Numerical simulation of heat production potential from hot dry rock by water circulating through a novel single vertical fracture at Desert Peak geothermal field | |
| Zhang et al. | Effect of reservoir’s permeability and porosity on the performance of cellular development model for enhanced geothermal system | |
| Bataillé et al. | Modeling the coupling between free and forced convection in a vertical permeable slot: Implications for the heat production of an Enhanced Geothermal System | |
| Aliyu et al. | Enhanced geothermal system modelling with multiple pore media: Thermo-hydraulic coupled processes | |
| CN104765973B (en) | Method for numerical simulation under the conditions of a kind of coal bed gas is adopted | |
| Yuan et al. | The numerical simulation of thermal recovery considering rock deformation in shale gas reservoir | |
| Gunnarsson et al. | Model simulations of the Hengill area, Southwestern Iceland | |
| Du et al. | Experimental and numerical simulation research on heat transfer performance of coaxial casing heat exchanger in 3500m-deep geothermal well in Weihe Basin | |
| Yin et al. | Analysis of flow and thermal breakthrough in leaky downhole coaxial open loop geothermal system | |
| Lv et al. | Analysis of heat transfer based on complex Embedded Discrete Fracture Network (EDFN) for field-scale EGS | |
| Zhang et al. | Effects of the spatial heterogeneity in reservoir parameters on the heat extraction performance forecast based on a 3D thermo-hydro-mechanical coupled model: A case study at the Zhacang geothermal field in the Guide basin, northeastern Tibetan plateau | |
| Gao et al. | A novel strategy utilizing local fracture networks to enhance CBHE heat extraction performance: A case study of the Songyuan geothermal field in China | |
| CN116341195A (en) | Deep crack-bedrock interbedded dry hot rock heat energy exploitation method | |
| Sun et al. | Prediction and optimization of productivity and lifespan in multi-well enhanced geothermal system | |
| Freeman et al. | THM Modeling for Reservoir Geomechanical Applications | |
| Gao et al. | Numerical simulation study of a novel horizontally layered enhanced geothermal system: A case study of the Qiabuqia geothermal area, Qinghai Province, China | |
| Aliyu et al. | Numerical modelling of geothermal reservoirs with multiple pore media | |
| Zhou et al. | Simulation of thermal transport in aquifer: a GWHP system in Chengdu, China | |
| Lee | Modeling on the cyclic operation of standing column wells under regional groundwater flow | |
| Lee | Performance of open borehole thermal energy storage system under cyclic flow regime | |
| Shen et al. | Study on the mechanism of water and heat transfer in sandstone geothermal system: a case study of doublet well | |
| Shang et al. | Analytical solutions of fractal-hydro-thermal model for two-phase flow in thermal stimulation enhanced coalbed methane recovery | |
| CN118376118B (en) | Mine water heat storage device and method for reconstructing abandoned coal mine shaft | |
| Wang et al. | Study on the production law of heavy oil gravity‐drainage‐assisted steam flooding |
Legal Events
| Date | Code | Title | Description |
|---|---|---|---|
| PB01 | Publication | ||
| PB01 | Publication | ||
| SE01 | Entry into force of request for substantive examination | ||
| SE01 | Entry into force of request for substantive examination |